TYPOLOGY OF OUR EDUCATIONAL MODELS

2. Explore

The key feature of a dynamic drawing is that, unlike ordinary paper drawings, it represents an infinite family of geometric figures rather than an individual figure. We can study this family by manipulating the initial elements of the construction that produces the family; this leads to a variety of activities some of which are described below.

2.1. Making a Geometric Discovery

Let's construct a triangle and the bisector of one of its angles. Now let's vary the triangle. It's no surprise that the ray we've constructed will stay a bisector no matter how we drag the triangle's vertices: after all, it was "born" to be a bisector. But if we draw the other two bisectors, we'll notice that all three bisectors meet at a common point and this concurrence persists even while the triangle is arbitrarily deformed. The surprising thing is we did not plan that the bisectors would concur! In this way, a variable construction allows us to make our own small geometric discovery.

Such a discovery can dramatically change the course of the lesson: from a melancholic presentation of "facts," even though accompanied by "passive" illustrations, the teacher moves to active stimulation of the students' creative potential. This fosters their ability to see, formulate, and understand geometric relationships and increasing their emotional involvement in learning.

Here is a more sophisticated example of this sort:

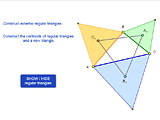Napoleon's Theorem

2.2. Numeric Experimentation

MathKit can measure lengths, angles, and areas. Although this feature "undermines" a whole class of traditional geometric problems, on the other hand, it extends the area of experiments and discoveries to numeric relationships.

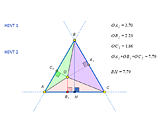The Sum of Distances to the Sides of a Regular Triangle

2.3. Opening a "Black Box"

Even a simple construction can be turned into an intriguing poser if you leave visible only some of its initial and some of its final elements ("input" and "output") and ask students to explain the relationship between them. This kind of activity, called a "black box problem," can be used just for fun, bulding student's creativity and imagination, but also it can be quite meaningful mathematically. For instance, in the example below one has to specify the isometry that maps one given figure on another one.

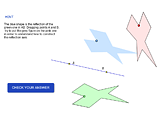Find the Transformation

2.4. Choosing a Proper Viewpoint

Here we present the type of activities that is one of the most popular among teachers. It is based on a three-dimensional construction that can be rotated around some axes, or, equivalently, that can be viewed from different directions. Such models have many different applications. In this particular case, the viewpoint must be chosen so as to see a certain plane as a line, which makes the solution to the problem in question almost obvious.

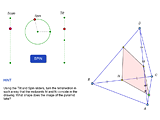Two-Midpoint Section of a Tetrahedron

2.5. Going to Extremes

The variability of dynamic models makes it feasible to investigate boundary and extremal situations. Suppose for example that you have constructed a triangle from its three given sides. Then you start to change their lengths, and the triangle suddenly disappears. This naturally brings you to an important question about the condition under which a triangle with given side lengths exists and the number of solutions depending on the initial data.

The example below presents the famous Heron Problem about the shortest path that starts at a given point, then hits a given line, and then arrives at another given point on the same side of the line as the first one. Students will try to find the solution by numeric experiment, and if it doesn't help, they can use hints.

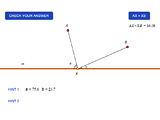Heron's Problem

2.6. Exploring Loci

MathKit provides means to construct and explore loci. This can be done with a tool that draws the bitmap trace of an object moving on the screen. But much more information can be obtained using the Locus tool. The loci it creates are dynamic curves similar to lines or circles in that one can take points on them, construct their intersection points with other curves, and observe their variation as their parent objects vary.

The example below models the well-known problem about a kitten on a ladder sliding down a wall. The model shows the path of a point ("kitten") on a segment of fixed length ("ladder") whose endpoints slide on the sides of a right angle. You can see that this path is an ellipse and trace its evolution as the position of the "kitten" changes. When the "kitten" sits at the midpoint, the ellipse becomes a circle, which is not difficult to prove.

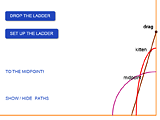Kitten on a Ladder

2.7. Exploring Parametric Equations

MathKit can plot functions depending on a variable parameter. This feature proves to be especially useful in solving algebraic equations and inequalities with parameters, which have become indispensable in Russian school curriculum and university exams in the last few years. By way of an example, we return to the equation considered in 1.3, replacing the bases of the logarithmic and exponential functions in it by an arbitrary numeric parameter.

Explore the dependence of the number of solutions on a parameter

The model allows you to make a qualitative analysis of the dependence of the number of solutions on the parameter a and determine approximately the critical values of a at which this number changes. To find these values exactly is another problem in which the computer is just an aid (and this is only good for teaching!).

Previous page	TYPOLOGY OF EDUCATIONAL MODELS	Next page